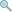Thermodynamics of irreversible phenomena.

> https://moodleucl.uclouvain.be/enrol/index.php?id=6793

• Elaboration of a general theoretical framework of irreversible phenomena having as starting points the kinetic theory of gases and classical thermodynamics
• Presentation of the classical theory of Onsager-Prigogine. Presentation of more recent theories such as Rational Thermodynamics (theory of Truesdell & Noll) and Extended Thermodynamics (theories of Jou & Lebon and of Müller).

With respect to the reference  AA of the programme of studies "Masters degree in Mechanical Engineering", this course contributes to the development and acquisition of the following skills

• AA1.1, AA1.2, AA1.3
• AA2.1, AA2.2, AA2.3
• AA3.1, AA3.3
• AA5.1, AA5.2, AA5.6
• AA6.1, AA6.2, AA6.3, AA6.4

Specific learning outcomes of the course

• A modern approach to non-equilibrium thermodynamics.
• Unified description of thermal, mechanical, viscous, and electromechanical processes in order to enchance the student's synthetic skills.
• Application of theoretical results in the modelling of irreversible phenomena in fluid and solid mechanics, geophysics, etc.

The contribution of this Teaching Unit to the development and command of the skills and learning outcomes of the programme(s) can be accessed at the end of this sheet, in the section entitled “Programmes/courses offering this Teaching Unit”.

Written exam, with open books and notes. The score on the course will be determined solely on the score on the exam.

• Course lectures
• Session of exercises

1. Kinetic approach. Presentation of the Maxwell-Boltzmann kinetic theory of gases. Relations between mascroscopic variables and kinetic theory. Derivation of principal transport coefficients (viscosity coefficient, conductivity, diffusivity), state equations, thermodynamic functions and their derivatives (internal energy, specific heats, entropy). Limits of continuum theory (rarefied gases, plasma). Study of specific problems in liquids (macromolecules) and solids (plasticity).
2. Continuum approach. Summary of equilibrium thermodynamics: first thermodynamic axiom (principle of energy conservation), absolute temperature and entropy, second thermodynamic axiom, thermodynamic potentials, thermochemistry and electrochemistry, Gibbs relations, equation of Gibbs & Duhem, phase transitions, interfaces.
3. Classical theory of irreversible thermodynamics (linear theory of Onsager-Prigogine): local equilibrium, entropy production, thermodynamic fluxes and forces, reciprocal relations, evolution laws and constitutive relations. Stationary states: criteria for minimum of entropy production and minimum of dissipated energy. Couplings between thermal, mechanical, and electromagnetic phenomena: thermoelectric and thermomagnetic effects.
4. Introduction to modern theories. Rational thermodynamics: material memory, objectivity, Clasius-Duhem inequality, constitutive relations. Applications in Non-Newtonian fluids and viscoelastic materials. Extended irreversible thermodynamics: basic hypotheses, causality, application in thermal conduction, second sound, comparison with the linear theory of Onsager-Prigogine.

• Lecture notes of the course LMECA2771 (in French). Recommended, available on the moodle site of the course.
• Supplementary notes on the kinetic theory of gases, thermoelectric phenomena and rational thermodynamics. Compulsory, available on the moodle site of the course.
• G. Lebon, D. Jou & J. Casas-Vasquez, Understanding Non-equilibrium Thermodynamics, Springer, 2008. Recommended.
• D. Kondepudi & I. Prigogine, Modern Thermodynamics, Wiley, 1999. Recommended.
• S.R. De Groot and P. Mazur, Non-equilibrium Thermodynamics, Dover, 1984. Recommended.